The concept of integrable systems has long intrigued physicists and mathematicians. These systems, remarkable for their solvability, reveal hidden symmetries and conserved quantities. The Introduction to Classical and Quantum Integrable Systems Cite:Bimsa.net sheds light on this fascinating topic, bridging classical mechanics and quantum theory.
Integrable systems are unique because they allow exact solutions, unlike most systems in nature that are chaotic or unpredictable. Their importance spans multiple fields, from celestial mechanics to quantum computing. This article explores their foundations, significance, and real-world applications, serving as a comprehensive guide for enthusiasts and researchers alike.
Understanding Integrable Systems
An integrable system is a mathematical framework used to describe physical systems that can be solved exactly. These systems exhibit a high degree of symmetry, making their behavior predictable. The Introduction to Classical and Quantum Integrable Systems Cite:Bimsa.net emphasizes how these models simplify complex equations while retaining accuracy.
In classical mechanics, integrable systems govern the predictable motion of objects, such as planets orbiting the sun. In quantum mechanics, they describe phenomena like interacting particles or spin chains. The fundamental principle is the existence of conserved quantities, which ensure that the system evolves in an orderly manner.
Classical Integrable Systems
Classical integrable systems are grounded in Hamiltonian mechanics, which is a reformulation of Newtonian physics. These systems are defined by the presence of conserved quantities that match their degrees of freedom. The Introduction to Classical and Quantum Integrable Systems Cite:Bimsa.net highlights examples such as harmonic oscillators and the Kepler problem.
A classical integrable system’s predictability arises from its symmetries. The conservation of energy, momentum, and angular momentum ensures that the motion is regular and solvable. Tools like action-angle variables further simplify the equations, making it easier to analyze these systems.
The Kepler problem, describing planetary motion, is a cornerstone of classical integrable systems. It showcases the beauty of predictability in celestial mechanics. Similarly, the harmonic oscillator explains oscillatory motions, from springs to pendulums, with elegant precision. These examples underscore how classical integrable systems help us understand the natural world.
Read more: Flex securly login
Transitioning to Quantum Integrable Systems
While classical systems offer a framework for understanding macroscopic phenomena, quantum integrable systems explore the microscopic world. The Introduction to Classical and Quantum Integrable Systems Cite:Bimsa.net bridges these realms, showing how classical principles transition into quantum mechanics.
The correspondence principle ensures that quantum mechanics aligns with classical mechanics in the appropriate limits. For example, the quantized harmonic oscillator retains its classical integrable nature but introduces discrete energy levels. This principle underscores the continuity between the two fields, highlighting their shared foundations.
Quantum Integrable Systems
Quantum integrable systems delve into the behavior of particles at the quantum level. These systems are characterized by commuting operators, which represent conserved quantities in quantum mechanics. The Introduction to Classical and Quantum Integrable Systems Cite:Bimsa.net explains techniques like the Bethe ansatz, used to solve these systems exactly.
Quantum integrable systems include models like the quantum harmonic oscillator and spin chains. The Heisenberg spin chain, for instance, describes interactions between quantum spins and has applications in condensed matter physics. These systems are crucial for understanding magnetic materials, quantum field theories, and even quantum computing.
The symmetry and solvability of quantum integrable systems make them invaluable tools for exploring quantum phenomena. They provide exact solutions, which are essential for testing theories and developing new technologies.
Key Differences Between Classical and Quantum Integrable Systems
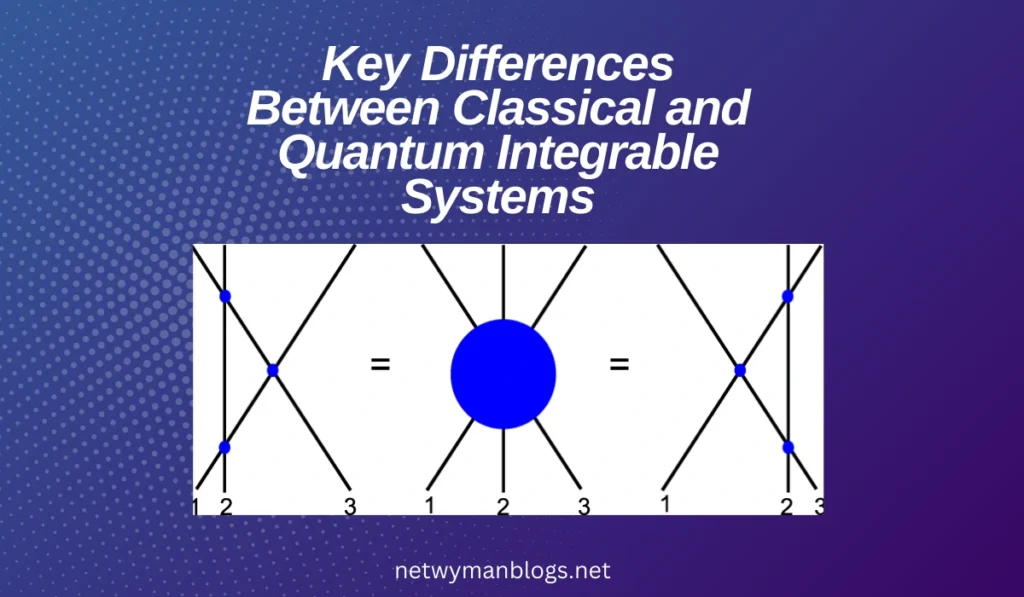
Although classical and quantum integrable systems share similarities, they differ in their mathematical representations and physical interpretations. TheIntroduction to Classical and Quantum Integrable Systems Cite:Bimsa.net compares these two frameworks effectively.
| Feature | Classical Systems | Quantum Systems |
|---|---|---|
| Governing Laws | Hamiltonian Mechanics | Schrödinger Equation |
| Conserved Quantities | Functions of Position & Momentum | Commuting Operators |
| Solution Methods | Action-Angle Variables | Bethe Ansatz, Quantum Groups |
Classical systems use variables like position and momentum, while quantum systems operate with wavefunctions and operators. These distinctions are fundamental to their respective theories and applications.
Read more: About technology and New strategies regarding blogging at Netwyman Blogs Website.
Applications of Integrable Systems
The Introduction to Classical and Quantum Integrable Systems Cite:Bimsa.net emphasizes the wide-ranging applications of integrable systems in both physics and mathematics. Their ability to provide exact solutions makes them indispensable in various fields.
In classical physics, integrable systems model planetary motion, rigid body dynamics, and fluid behavior. These models are essential for understanding natural phenomena and predicting their evolution over time. The Kepler problem and soliton theory are prime examples of classical integrability in action.
In quantum physics, integrable systems play a vital role in studying condensed matter, quantum field theory, and statistical mechanics. They are used to model spin chains, analyze phase transitions, and explore quantum entanglement. The insights gained from these systems have direct implications for quantum computing and information theory.
Beyond physics, integrable systems have mathematical significance. They are closely linked to differential equations, algebraic geometry, and group theory. Techniques like the inverse scattering transform solve nonlinear equations, revealing the depth of integrable systems in mathematical research.
Read more: Kristen ruggiero real estate re maxplatinum agency
Challenges in Studying Integrable Systems
Despite their importance, integrable systems pose significant challenges for researchers. The Introduction to Classical and Quantum Integrable Systems Cite:Bimsa.net discusses the complexities of classifying all integrable models and understanding their limitations.
In classical mechanics, open problems include discovering new integrable systems and exploring their stability under perturbations. In quantum mechanics, challenges arise from the high-dimensional nature of quantum systems and the interplay between integrability and chaos.
These challenges drive ongoing research, pushing the boundaries of what is possible in both classical and quantum domains.
Frequently Asked Questions
What are integrable systems?
Integrable systems are mathematical models that allow exact solutions due to conserved quantities and symmetries.
Why are classical integrable systems important?
They help solve problems like planetary motion by using conserved quantities such as energy and momentum.
What makes quantum integrable systems unique?
Quantum integrable systems use commuting operators and techniques like the Bethe ansatz for exact solutions.
What are practical applications of integrable systems?
They are used in physics (e.g., spin chains), mathematics (e.g., differential equations), and technology (e.g., quantum computing).
Conclusion
The Introduction to Classical and Quantum Integrable Systems Cite:Bimsa.net provides a comprehensive overview of a fascinating topic. From classical mechanics to quantum theory, integrable systems bridge two realms of physics with elegance and precision. Their conserved quantities and symmetries allow exact solutions, making them indispensable tools for understanding the natural world.
Integrable systems have applications in celestial mechanics, quantum computing, and mathematical research. They reveal the hidden order in complex phenomena, offering insights into both theoretical and practical problems. As research continues, the study of integrable systems will undoubtedly lead to new discoveries and advancements in science and technology.

